The Riemann hypothesis is the most important open question in number theory—if not all of mathematics. It has occupied experts for more than 160 years. And the problem appeared both in mathematician David Hilbert’s groundbreaking speech from 1900 and among the “Millennium Problems” formulated a century later. The person who solves it will win a million-dollar prize.
But the Riemann hypothesis is a tough nut to crack. Despite decades of effort, the interest of many experts and the cash reward, there has been little progress. Now mathematicians Larry Guth of the Massachusetts Institute of Technology and James Maynard of the University of Oxford have posted a sensational new finding on the preprint server arXiv.org. In the paper, “the authors improve a result that seemed insurmountable for more than 50 years,” says number theorist Valentin Blomer of the University of Bonn in Germany.
Other experts agree. The work is “a remarkable breakthrough,” mathematician and Fields Medalist Terence Tao wrote on Mastodon, “though still very far from fully resolving this conjecture.”
On supporting science journalism
If you’re enjoying this article, consider supporting our award-winning journalism by subscribing. By purchasing a subscription you are helping to ensure the future of impactful stories about the discoveries and ideas shaping our world today.
The Riemann hypothesis concerns the basic building blocks of natural numbers: prime numbers, values only divisible by 1 and themselves. Examples include 2, 3, 5, 7, 11, 13, and so on.
Every other number, such as 15, can be clearly broken down into a product of prime numbers: 15 = 3 x 5. The problem is that the prime numbers do not seem to follow a simple pattern and instead appear randomly among the natural numbers. Nineteenth-century German mathematician Bernhard Riemann proposed a way to deal with this peculiarity that explains how prime numbers are distributed on the number line—at least from a statistical point of view.
A Periodic Table for Numbers
Proving this conjecture would provide mathematicians with nothing less than a kind of “periodic table of numbers.” Just as the basic building blocks of matter (such as quarks, electrons and photons) help us to understand the universe and our world, prime numbers also play an important role, not just in number theory but in almost all areas of mathematics.
There are now numerous theorems based on the Riemann conjecture. Proof of this conjecture would prove many other theorems as well—yet another incentive to tackle this stubborn problem.
Interest in prime numbers goes back thousands of years. Euclid proved as early as 300 B.C.E. that there are an infinite number of prime numbers. And although interest in prime numbers persisted, it was not until the 18th century that any further significant findings were made about these basic building blocks.
As a 15-year-old, physicist Carl Friedrich Gauss realized that the number of prime numbers decreases along the number line. His so-called prime number theorem (not proven until 100 years later) states that approximately n/ln(n) prime numbers appear in the interval from 0 to n. In other words, the prime number theorem offers mathematicians a way of estimating the typical distribution of primes along a chunk of the number line.
The exact number of prime numbers may differ from the estimate given by the theorem, however. For example: According to the prime number theorem, there are approximately 100/ln(100) ≈ 22 prime numbers in the interval between 1 and 100. But in reality there are 25. There is therefore a deviation of 3. This is where the Riemann hypothesis comes in. This hypothesis gives mathematicians a way to estimate the deviation. More specifically, it states that this deviation cannot become arbitrarily large but instead must scale at most with the square root of n, the length of the interval under consideration.
The Riemann hypothesis therefore does not predict exactly where prime numbers are located but posits that their appearance on the number line follows certain rules. According to the Riemann hypothesis, the density of primes decreases according to the prime number theorem, and the primes are evenly distributed according to this density. This means that there are no large areas in which there are no prime numbers at all, while others are full of them.
You can also imagine this idea by thinking about the distribution of molecules in the air of a room: the overall density on the floor is somewhat higher than on the ceiling, but the particles—following this density distribution—are nonetheless evenly scattered, and there is no vacuum anywhere.
A Strange Connection
Riemann formulated the conjecture named after him in 1859, in a slim, six-page publication (his only contribution to the field of number theory). At first glance, however, his work has little to do with prime numbers.
He dealt with a specific function, the so-called zeta function ζ(s), an infinitely long sum that adds the reciprocal values of natural numbers that are raised to the power of s:
Even before Riemann’s work, experts knew that such zeta functions are related to prime numbers. Thus, the zeta function can also be expressed as a function of all prime numbers p as follows:

Riemann recognized the full significance of this connection with prime numbers when he used not only real values for s but also complex numbers. These numbers contain both a real part and roots from negative numbers, the so-called imaginary part.
You can imagine complex numbers as a two-dimensional construct. Rather than mark a point on the number line, they instead lie on the plane. The x coordinate corresponds to the real part and the y coordinate to the imaginary part:
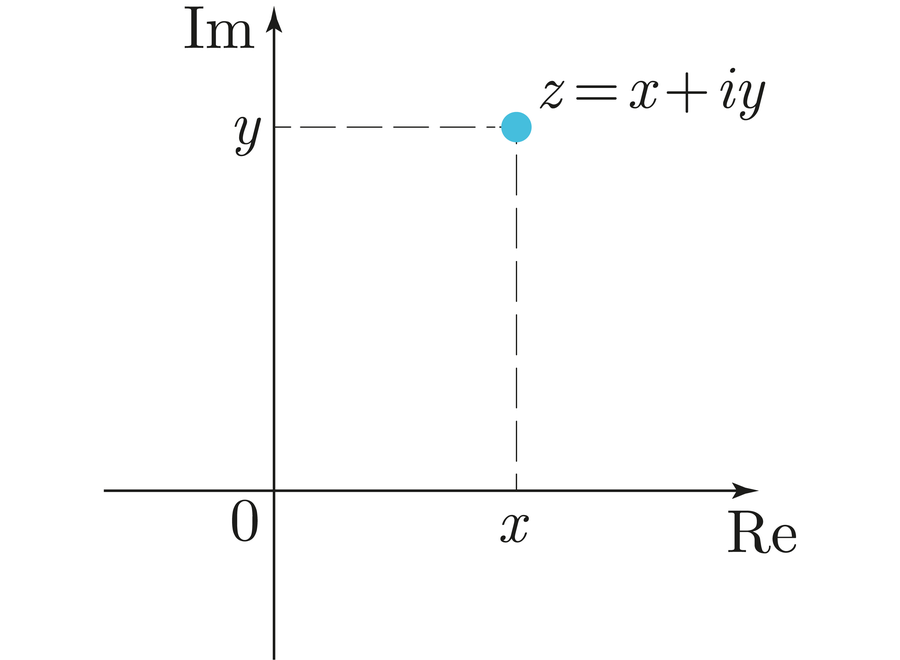
Никита Воробьев/Wikimedia
The complex zeta function that Riemann investigated can be visualized as a landscape above the plane. As it turns out, there are certain points amid the mountains and valleys that play an important role in relation to prime numbers. These are the points at which the zeta function becomes zero (so-called zeros), where the landscape sinks to sea level, so to speak.
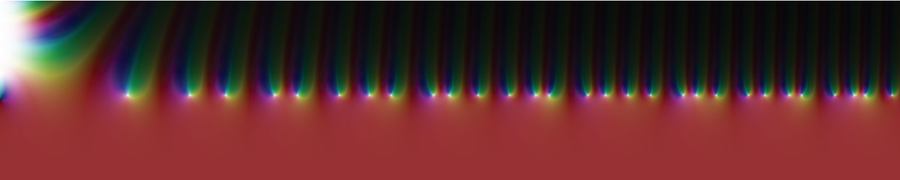
The colors represent the values of the complex zeta function, with the white dots indicating its zeros.
Riemann quickly found that the zeta function has no zeros if the real part is greater than 1. This means that the area of the landscape to the right of the straight line x = 1 never sinks to sea level. The zeros of the zeta function are also known for negative values of the real part. They lie on the real axis at x = –2, –4, –6, and so on. But what really interested Riemann—and all mathematicians since—were the zeros of the zeta function in the “critical strip” between 0 ≤ x ≤ 1.
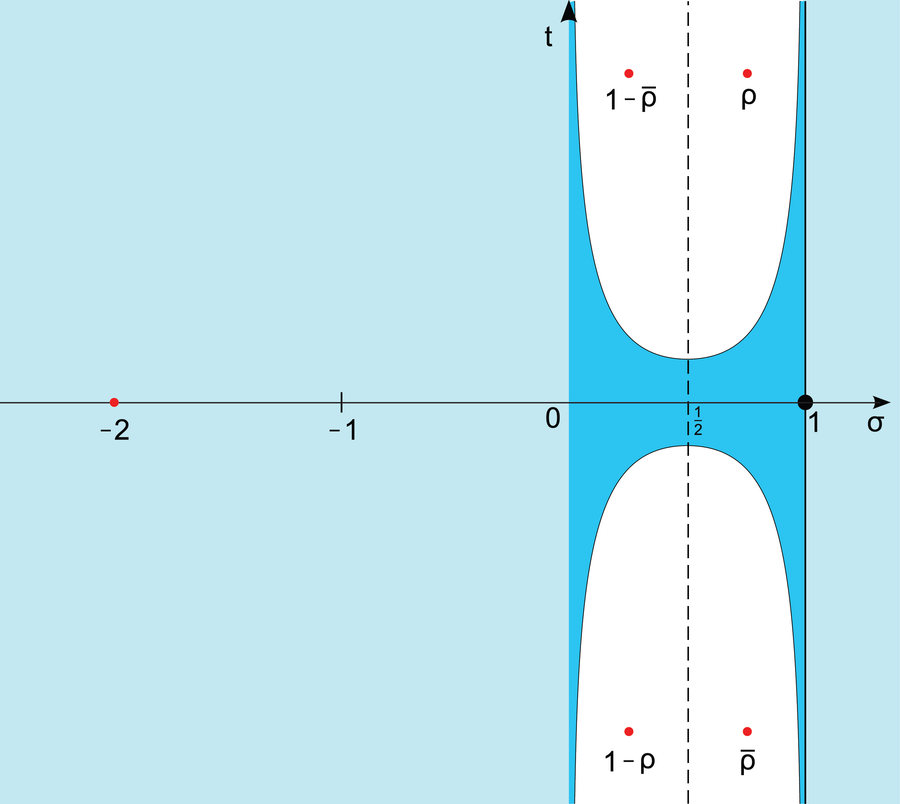
In the critical strip (dark blue), the Riemann zeta function can have “nontrivial” zeros. The Riemann conjecture states that these are located exclusively on the line x = 1/2 (dashed line).
Riemann knew that the zeta function has an infinite number of zeros within the critical strip. But interestingly, all appear to lie on the straight line x = 1/2. Thus Riemann hypothesized that all zeros of the zeta function within the critical strip have a real part of x = 1/2. That statement is actually at the crux of understanding the distribution of prime numbers. If correct, then the placement of prime numbers along the number line never deviates too much from the prime number set.
On the Hunt for Zeros
To date, billions and billions of zeta function zeros have now been examined—more than 1013 of them—and all lie on the straight line x = 1/2.
But that alone is not a valid proof. You would only have to find a single zero that deviates from this scheme to disprove the Riemann hypothesis. Therefore we are looking for a proof that clearly demonstrates that there are no zeros outside x = 1/2 in the critical strip.
Thus far, such a proof has been out of reach, so researchers took a different approach. They tried to show that there is, at most, a certain number N of zeros outside this straight line x = 1/2. The hope is to reduce N until N = 0 at some point, thereby proving the Riemann conjecture. Unfortunately, this path also turns out to be extremely difficult. In 1940 mathematician Albert Ingham was able to show that between 0.75 ≤ x ≤ 1 there are at most y3/5+c zeros with an imaginary part of at most y, where c is a constant between 0 and 9.
In the following 80 years, this estimation barely improved. The last notable progress came from mathematician Martin Huxley in 1972. “This has limited us from doing many things in analytic number theory,” Tao wrote in his social media post. For example, if you wanted to apply the prime number theorem to short intervals of the type [x, x + xθ], you were limited by Ingham’s estimate to θ > 1/6.
Yet if Riemann’s conjecture is true, then the prime number theorem applies to any interval (or θ = 0), no matter how small (because [x, x + xθ] = [x, x + 1] applies to θ = 0).
Now Maynard, who was awarded the prestigious Fields Medal in 2022, and Guth have succeeded in significantly improving Ingham’s estimate for the first time. According to their work, the zeta function in the range 0.75 ≤ x ≤ 1 has at most y(13/25)+c zeros with an imaginary part of at most y. What does that mean exactly? Blomer explains: “The authors show in a quantitative sense that zeros of the Riemann zeta function become rarer the further away they are from the critical straight line. In other words, the worse the possible violations of the Riemann conjecture are, the more rarely they would occur.”
“This propagates to many corresponding improvements in analytic number theory,” Tao wrote. It makes it possible to reduce the size of the intervals for which the prime number theorem applies. The theorem is valid for [x, x + x2/15], so θ > 1/6 = 0.166… becomes θ > 2⁄15 = 0.133…
For this advance, Maynard and Guth initially used well-known methods from Fourier analysis for their result. These are similar techniques to what is used to break down a sound into its overtones. “The first few steps are standard, and many analytic number theorists, including myself, who have attempted to break the Ingham bound, will recognize them,” Tao explained. From there, however, Maynard and Guth “do a number of clever and unexpected maneuvers,” Tao wrote.
Blomer agrees. “The work provides a whole new set of ideas that—as the authors rightly say—can probably be applied to other problems. From a research point of view, that’s the most decisive contribution of the work,” he says.
So even if Maynard and Guth have not solved Riemann’s conjecture, they have at least provided new food for thought to tackle the 160-year-old puzzle. And who knows—perhaps their efforts hold the key to finally cracking the conjecture.
This article originally appeared in Spektrum der Wissenschaft and was reproduced with permission.



















Discussion about this post